Another year has flown by here at Wolfram|Alpha, and the gears are really turning! New data and features are flowing at a rapid rate. To celebrate, Wolfram|Alpha’s creator, Stephen Wolfram, will share what we’ve been working on and take your questions in a live Q&A.

Please join us on Facebook or Wolfram|Alpha’s Livestream on Wednesday, May 18, 2011, at 10am PDT/12pm CDT/1pm EDT/6pm BST.

If you have a question you’d like to ask, please send it as a comment to this blog post or tweet to @Wolfram_Alpha and include the hashtag #WAChat. We’ll also be taking questions live on Facebook and Livestream chat during the webcast.

We’re looking forward to chatting with you on May 18!

Do you need some help navigating your chemistry or precalculus classes? Or maybe you’re still trying to decide which classes to take this fall. Good news! Today, we’re releasing the Wolfram General Chemistry and Precalculus Course Assistant Apps, two more Wolfram|Alpha-powered course assistants that will help you better understand the concepts addressed in these classes.

If you’re taking chemistry, download the Wolfram General Chemistry Course Assistant App for everything from looking up simple properties like electron configurations to computing the stoichiometric amounts of solutes that are present in solutions of different concentrations. This app is handy for lab researchers, too!

The specialized keyboard allows you to enter chemicals by using formulas or by spelling out their names.
More »

Today we are releasing Wolfram Multivariable Calculus and Wolfram Astronomy, the next two apps on a growing list of Wolfram Course Assistant Apps. These course assistants will help students learn their course material using the power of Wolfram|Alpha.

The Wolfram Astronomy Course Assistant allows you to easily look up information on constellations and planets, but it can also calculate anything from the next lunar eclipse to the solar interior.

More »

Today we’re releasing the first three of a planned series of “course assistant” apps, built using Wolfram|Alpha technology.

The long-term goal is to have an assistant app for every major course, from elementary school to graduate school. And the good news is that Wolfram|Alpha has the breadth and depth of capabilities to make this possible—and not only in traditionally “computational” kinds of courses.

The concept of these apps is to make it as quick and easy as possible to access the particular capabilities of Wolfram|Alpha relevant for specific courses. Each app is organized according to the major curriculum units of a course. Then within each section of the app, there are parts that cover each of the particular types of problems relevant to that unit.
More »

A new school year is here, and many students are diving into new levels of math. Fortunately, this year, you have Wolfram|Alpha to help you work through math problems and understand new concepts. Wolfram|Alpha contains information from the most basic math problems to advanced and even research-level mathematics. If you are not yet aware of Wolfram|Alpha’s math capabilities, you are about to have a “wow” moment. For the Wolfram|Alpha veterans, we have added many math features since the end of the last school year. In this post, we’re highlighting some existing Wolfram|Alpha math essentials, such as adding fractions, solving equations, statistics, and examples from new topics areas like cusps and corners, stationary points, asymptotes, and geometry.

You can access the computational power of Wolfram|Alpha through the free website, via Wolfram|Alpha Widgets, with the Wolfram|Alpha App for iPhone, iPod touch, and the iPad! Even better, the Wolfram|Alpha Apps for iPhone, and iPod touch, and the iPad are now on sale in the App Store for $0.99 though September 12.

If you need to brush up on adding fractions, solving equations, or finding a derivative, Wolfram|Alpha is the place to go. Wolfram|Alpha not only has the ability to find the solutions to these math problems, but also to show one way of reaching the solution with the “Show Steps” button. Check out the post “Step-by-Step Math” for more on this feature.

You can find this widget, and many others, in the Wolfram|Alpha Widget Gallery. Customize or build your own to help you work through common math problems. Then add these widgets to your website or blog, and share them with friends on Facebook and other social networks.

Of course, Wolfram|Alpha also covers statistics and probability. For example, Wolfram|Alpha can compute coin tossing probabilities such as “probability of 21 coin tosses“, and provides information on normal distribution: More »

Wolfram|Alpha computes things. While the use of computations to predict the outcomes of scientific experiments, natural processes, and mathematical operations is by no means new (it has become a ubiquitous tool over the last few hundred years), the ease of use and accessibility of a large, powerful, and ever-expanding collection of such computations provided by Wolfram|Alpha is.

Virtually all known processes occur in such a way that certain functionals that describe them become extremal. Typically this happens with the action for time dependent processes and quantities such as the free energy for static configurations. The equations describing the extremality condition of a functional are frequently low-order ordinary and/or partial differential equations and their solutions. For example, for a pendulum: Frechet derivative of Integrate[x'[t]^2/2 – Cos[x[t]], {t, -inf, inf}] wrt x[tau]. Unfortunately, if one uses a sufficiently realistic physical model that incorporates all potentially relevant variables (including things like friction, temperature dependence, deformation, and so forth), the resulting equations typically become complicated—so much so that in most cases, no exact closed-form solution can be found, meaning the equations must be solved using numerical techniques. A simple example is provided by free fall from large heights:

On the other hand, some systems, such as the force of a simple spring, can be described by formulas involving simple low-order polynomial or rational relations between the relevant problem variables (in this case, Hooke’s law, F = k x):

Over the last 200+ years, mathematicians and physicists have found a large, fascinating, and insightful world of phenomena that can be described exactly using these so-called special functions (also commonly known as “the special functions of mathematical physics”), the class of functions that describe phenomena between being difficult and complicated. It includes a few hundred members, and can be viewed as an extension of the so-called elementary functions such as exp(z), log(z), the trigonometric functions, their inverses, and related functions.

Special functions turn up in diverse areas ranging from the spherical pendulum in mechanics to inequivalent representations in quantum field theory, and most of them are solutions of first- or second-order ordinary differential equations. Textbooks often contain simple formulas that correspond to a simplified version of a general physical system—sometimes even without explicitly stating the implicit simplifying assumptions! However, it is often possible to give a more precise and correct result in terms of special functions. For instance, many physics textbooks offer a simple formula for the inductance of a circular coil with a small radius:

While Wolfram|Alpha knows (and allows you to compute with) this simple formula, it also knows the correct general result. In fact, if you just ask Wolfram|Alpha for inductance circular coil, you will be simultaneously presented with two calculators: the one you know from your electromagnetics textbook (small-radius approximation) and the fully correct one. And not only can you compute the results both ways (and see that the results do differ slightly for the chosen parameters, but that the difference can get arbitrarily large), you can also click on the second “Show formula” link (near the bottom of the page on the right side) to see the exact result—which, as can be seen, contains two sorts of special functions, denoted E(m) and K(m) and known as elliptic integrals: More »

Exciting new math features have arrived in Wolfram|Alpha! Our programmers have spent the past two months developing new capabilities in optimization, probability, number theory, and a host of other mathematical disciplines. Searching for elusive extrema? Look no further! Just feed your function(s) into Wolfram|Alpha and ask for their maxima, minima, or both. You can find global maxima and minima, optimize a function subject to constraints, or simply hunt for local extrema.

We’ve also added support for a wide variety of combinatorics and probability queries. Counting combinations and generating binomial coefficients has been simplified with syntax like 30 choose 18. Want to spend less time crunching numbers and more time practicing your poker face? You can ask directly for the probability of a full house or other common hands, as well as the probabilities of various outcomes when you play Powerball, roll two 12-sided dice, or repeat any sequence of trials with a 20% chance 4 times.

The pursuit of primes has never been so simple. Imagine yourself walking the streets of an infinite city in search of “prime real estate.” You can find the nearest one simply by requesting (for example) the prime closest to 100854; alternatively, you could scope out the entire neighborhood by asking Wolfram|Alpha to list primes between 100,000 and 101,000. Would you prefer the greatest prime number with 10 digits, or will you be satisfied with any random prime between 100,000,000 and 200,000,000? The aspiring real estate agent—er, number theoretician—can also tinker with quantities like the sum of the first hundred primes or the product of primes between 900 and 1000. If your explorations take you to the realm of the composites (the addresses of houses with “sub-prime” mortgages, perhaps), you can identify numbers with shared factors by querying Wolfram|Alpha for, say, multiples of 5, 17, 21.

Other additions have brought everything from Archimedes’ axiom to semiaxes and square pyramid syntax into our body of computable knowledge and functions. Wolfram|Alpha grows daily, so stay tuned to this blog for further updates. Better yet, apply to become a Wolfram|Alpha tester for privileged access to the newest features before they go public!

Valentine’s Day is special to sweethearts around the world. While Wolfram|Alpha can’t come close to replacing a thoughtful card or gourmet box of chocolates, there are a surprisingly large number of things related to Valentine’s Day (and in particular, to its central icon) that Wolfram|Alpha can compute.

Let’s start with the holiday itself. Just typing in “valentine’s day” gives the expected calendrical information, from which we learn that Valentine’s Day falls on a Sunday this year. For the procrastinators among us, we can also find out how many days we have remaining to acquire an appropriate token of affection for our loved one (or by how many days we’ve already blown our chance). Wolfram|Alpha also shows various other useful data, including the interesting fact that Valentine’s Day coincides with Chinese New Year this year.

While Wolfram|Alpha can’t (yet) tell you how many calories are in your box of holiday chocolates or package of Valentine’s Day Sweethearts candy, there are plenty of computational objects related to that most-famous Valentine’s Day icon—the heart—that it can tell you something interesting and/or useful about. For instance, do you know the average weight of a human heart? The typical resting heart rate? The Unicode point for the heart symbol character? Or perhaps you’ve forgotten the ASCII keystrokes needed to insert a love emoticon at the end of an email to your Sweet Baboo?

On the mathematical side, typing in “heart curve” gives you a number of mathematical curves resembling the heart shape. The default (and probably most famous) of these is the cardioid, whose name after all means “heart-shaped” in Latin (and about which we all have fond memories dating back to our introductory calculus courses):

A curve more closely resembling the conventional schematic (if not physiological) heart shape is the so-called “first heart curve“, which is an algebraic curve described by a beautifully simple sextic Cartesian equation:

If you don’t care for any of the heart curves Wolfram|Alpha knows about (or even if you do), you’re also of course also free to experiment with your own. For example, a particularly attractive curve can be obtained using the relatively simple input “polar plot 2 – 2 sin t + sin t sqrt (abs(cos t))/(sin t + 1.4)“. More »

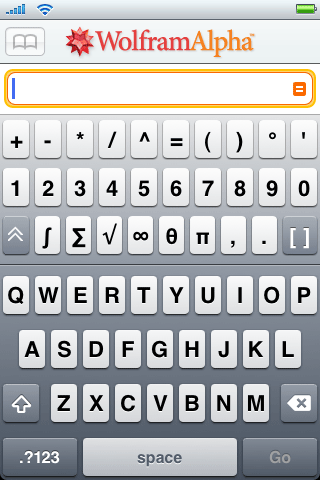
Version 1.1 of the Wolfram|Alpha App for the iPhone & iPod is now available in the App Store. The new version includes a number of new features that continue to improve the app’s unique mobile Wolfram|Alpha experience. Perhaps its most iconic feature, the specialized keyboards that greet you when you first open the Wolfram|Alpha App, have been painstakingly constructed to ease the burden of entering queries, whether you’re converting from pounds to euros or computing a numerical value for the Weierstrass p-function . Our goal in creating these keyboards was to form families of characters that naturally occur together both in common use and in traditional mathematical applications. We also wanted mathematical expressions to look and feel natural to enhance usability and understanding. Version 1.1 has four specialized keyboards: the default keyboard, the “math” keyboard accessed by the right-shift key  , the “Greek” keyboard accessed by one press of the left-shift key , and the “symbol” keyboard accessed by a second press of the left-shift key.

To determine the optimal keyboard layout, we scoured Wolfram|Alpha’s server logs for the most commonly entered phrases that have characters with meaning in Wolfram|Alpha. Given that Wolfram|Alpha is built on Mathematica, one of its core strengths is advanced mathematics. True to form most of the commonly typed characters are related to math. For example, you would generally type the word “integrate” to compute an integral on the Wolfram|Alpha website. In the Wolfram|Alpha App you could simply type the  key on the math keyboard. The same is true for other symbols common in math, such as and . Specifying geometric shapes, such as a triangle, is straightforward as well.

More »

Prior to releasing Wolfram|Alpha into the world this past May, we launched the Wolfram|Alpha Blog. Since our welcome message on April 28, we’ve made 133 additional posts covering Wolfram|Alpha news, team member introductions, and “how-to’s” in a wide variety of areas, including finance, nutrition, chemistry, astronomy, math, travel, and even solving crossword puzzles.

As 2009 draws to a close we thought we’d reach into the archives to share with you some of this year’s most popular blog posts.

April

Rack ’n’ Roll

Take a peek at our system administration team hard at work on one of the
many pre-launch projects. Continue reading…

May

The Secret Behind the Computational Engine in Wolfram|Alpha

Although it’s tempting to think of Wolfram|Alpha as a place to look up facts, that’s only part of the story. The thing that truly sets Wolfram|Alpha apart is that it is able to do sophisticated computations for you, both pure computations involving numbers or formulas you enter, and computations applied automatically to data called up from its repositories.

Why does computation matter? Because computation is what turns generic information into specific answers. Continue reading…

Live, from Champaign!

Wolfram|Alpha just went live for the very first time, running all clusters.

This first run at testing Wolfram|Alpha in the real world is off to an auspicious start, although not surprisingly, we’re still working on some kinks, especially around logging.

While we’re still in the early stages of this long-term project, it is really gratifying to finally have the opportunity to invite you to participate in this project with us. Continue reading…

June

Wolfram|Alpha Q&A Webcast

Stephen Wolfram shared the latest news and updates about Wolfram|Alpha and answered several users’ questions in a live webcast yesterday.

If you missed it, you can watch the recording here. Continue reading… More »

We’re really catching the holiday spirit here at Wolfram|Alpha.

We recently announced our special holiday sale for the Wolfram|Alpha app. Now we are launching our first-ever Wolfram|Alpha “Holiday Tweet-a-Day” contest.

Here’s how it works.

From tomorrow, Tuesday, December 22, through Saturday, January 2, we’ll use Twitter to give away a gift a day. Be the first to retweet our “Holiday Tweet-a-Day” tweet and you get the prize! You can double your chances to win by following and playing along with Wolfram Research.

Start following us today so you don’t miss your chance to win with our Wolfram|Alpha “Holiday Tweet-a-Day” contest.

When we launched Wolfram|Alpha in May 2009, it already contained trillions of pieces of information—the result of nearly five years of sustained data-gathering, on top of more than two decades of formula and algorithm development in Mathematica. Since then, we’ve successfully released a new build of Wolfram|Alpha’s codebase each week, incorporating not only hundreds of minor behind-the-scenes enhancements and bug fixes, but also a steady stream of major new features and datasets.

We’ve highlighted some of these new additions in this blog, but many more have entered the system with little fanfare. As we near the end of 2009, we wanted to look back at seven months of new Wolfram|Alpha features and functionality.

More »

July 17, 2019 Update: Step-by-step solutions has been updated! Learn more.

Have you ever given up working on a math problem because you couldn’t figure out the next step? Wolfram|Alpha can guide you step by step through the process of solving many mathematical problems, from solving a simple quadratic equation to taking the integral of a complex function.

When trying to find the roots of 3x²+x–7=4x, Wolfram|Alpha can break down the steps for you if you click the “Show steps” button in the Result pod.

As you can see, Wolfram|Alpha can find the roots of quadratic equations. Wolfram|Alpha shows how to solve this equation by completing the square and then solving for x. Of course, there are other ways to solve this problem! More »

We know college is hard. So we’re highlighting examples of how Wolfram|Alpha can make subjects and concepts a bit easier to learn. Wolfram|Alpha is a free computational knowledge engine that can help you tackle everything from calculus, to computing the number of pages for a double-spaced 1000-word essay, to comparing the flash points of methane, butane, and octane, to figuring just how much money it’s going to cost you to drive home to do your laundry. Check out a quick introduction to Wolfram|Alpha from its creator, Stephen Wolfram.

We want to help you take full advantage of this resource. Over the next term, we’ll be highlighting helpful computations and information here on the blog, and even providing ways you can get involved with our company. (Would you like to be a part of the Wolfram|Alpha Team on your campus? Stay tuned to find out how you can be involved.) For this post we selected several of our favorite examples to help you start thinking about how you can use Wolfram|Alpha in your courses, and in your always-changing college life. More »

We use this blog to provide helpful tips on using Wolfram|Alpha. So when a relevant screencast caught our eye on Twitter—“Wolfram|Alpha for Calculus Students,” produced by Robert Talbert, PhD, an associate professor of mathematics and computing science at Franklin College—we wanted share it with you. We think his straightforward video is a great demonstration of just how valuable Wolfram|Alpha is for students. In the screencast, Professor Talbert discusses the concept of Wolfram|Alpha, and illustrates how it solves problems such as factoring or expanding expressions, solving quadratic equations, and more.

The screencast covers just a few of the ways educators and students are using Wolfram|Alpha. Are you an instructor who has found innovative ways to incorporate Wolfram|Alpha into your lesson plans? Or are you a student using Wolfram|Alpha to assist in your studies? You can join others having these conversations on the Wolfram|Alpha Community site.

We’ve updated another entry thanks to feedback sent to Wolfram|Alpha. We’ve now changed linguistic priority settings so that “blog” is no longer interpreted as the math expression b log(x) by default.

More »

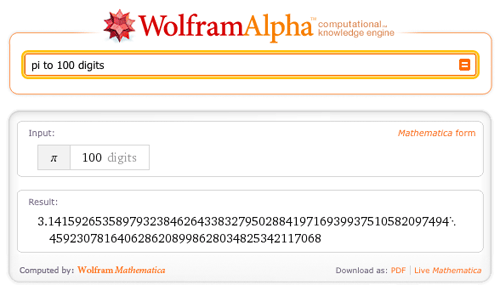
Some of you have asked whether you’ll be able to use Wolfram|Alpha for challenging math. Of course!

Remember your old friend pi?

More »