3
3
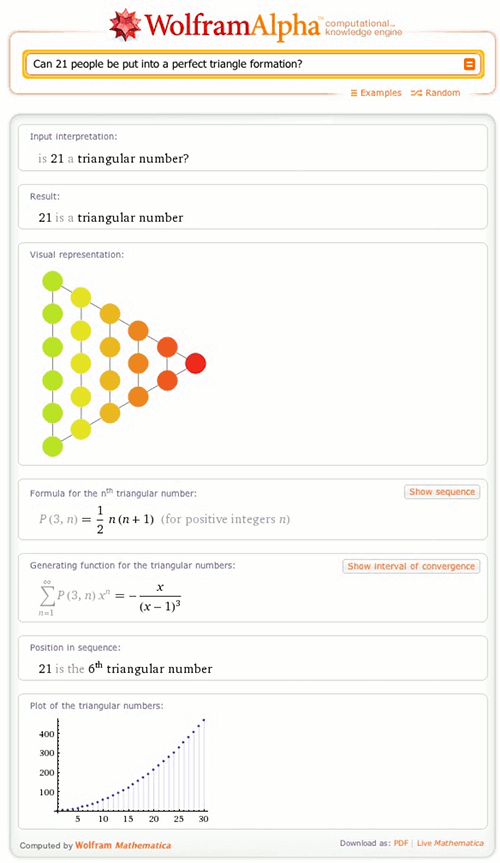
Want to know if you and your 20 teammates can march in perfect triangular formation in a parade? Or maybe you want to know how many glasses you’ll need in order to break the world record for largest champagne pyramid (currently 60 stories high, with 37,820 glasses).
Whether you’re stacking watermelons or water-skiers or just playing with dots on paper, if you want to make a perfect geometrical formation, then you’re interested in “figurate” numbers. These are numbers for which that number of things can be arranged into a perfect geometrical shape, such as a triangle (in the case of the “triangular numbers”) or a triangular pyramid (AKA, a tetrahedron), which is the shape of champagne pyramids.
Wolfram|Alpha now has the ability to tell you if a given number is any figurate number. Entering our first question, “Can 21 people be put into a perfect triangle formation?”, into Wolfram|Alpha gives an affirmative, along with a diagram and other useful information:
 4
4
Consider packing circles inside a circular container, or less abstractly, placing cookie dough on a cookie sheet. In the case of cookies, which expand to be a roughly circular shape, you don’t want them so close that they run into each other. At the same time, you don’t want them too far apart, because that would mean fewer cookies.
One of the latest features of Wolfram|Alpha is the ability to get information about packing circles into circles.
For instance, suppose you have a circular baking sheet with a diameter of 12 inches, and you want to make 20 cookies. You can ask Wolfram|Alpha “pack 20 circles in a diameter 12 inch circle”; not only does it give you a diagram of the densest packing, but also the largest radius of the circular cookies on the 12-inch baking sheet.
Or you might know the size of the cookies and want to know how many can fit? One way to get the answer would be “pack r=1 circles in a diameter 12 circle”.
More »
 2
2
Wolfram|Alpha already contains many extensive collections of mathematical data, including curves, surfaces, graphs, knots, and polyhedra. However, one type of object we had not systematically incorporated until recently was the class of plane geometric figures technically known as laminae:
Most people (including the subset of small people who play with sorting toys such as the one illustrated below) are familiar with a number of laminae. A lamina is simply a bounded (and usually connected) region of the Euclidean plane. In the most general case, it has a surface density function ?(x, y) as a function of x– and y-coordinates, but with ?(x, y) = 1 in the simplest case.

Examples of laminae, some of which are illustrated above, therefore include the disk (i.e., filled circle), equilateral triangle, square, trapezoid, and 5-point star. In the interest of completeness, it might be worth mentioning that laminae are always “filled” objects, so the ambiguity about whether the terms “polygon”, “square”, etc. refer to closed sets of line segments or those segments plus their interiors does not arise for laminae.
More »
 10
10
As always, we are striving for better linguistic support of all things math, and over the past few months, we have made many improvements to that end.
We have significantly improved our support for queries involving polygons and circles being inscribed in and circumscribed about each other. Give these examples a spin:
Another improvement of note is that we have gotten better accepting queries like “algebraically find the inverse function of f(x)=3-8e^x” and winnowing this down to the core question, that of “inverse of f(x)=3-8e^x”. More »
 7
7
Here at Wolfram|Alpha we’re always asking questions and seeking answers in an effort to make all of the world’s knowledge computable and understandable by everyone (big or small).
We’ve put together a short list of common questions asked by preschool- and kindergarten-aged children that can be answered with Wolfram|Alpha. We hope these examples inspire your child to dream up more!
Is the Moon bigger than the Earth? Ask Wolfram|Alpha to compare “size of earth, size of moon”, and you’ll discover numerical and graphic size comparisons showing that the Earth is indeed larger than the Moon.
Chances are your little artists will discover the answer to this question on their own, but they can try asking Wolfram|Alpha what color they get when they “mix red and blue”?
Whether it’s because they’re excited about the party or just turning a year older, the birthday countdown is always on! Simply ask Wolfram|Alpha about the date of the child’s upcoming birthday, such as “October 8 2010”, to learn the number of days, weeks, or months until the big day.
More »
 33
33
Every aspect of Wolfram|Alpha has been thought through in great detail. Its logo is no exception.
As a tip of the hat to the vast and powerful computational engine that powers Wolfram|Alpha, a natural place to start brainstorming for an appropriate logo was in Mathematica itself. And this is where I, geometry enthusiast and the developer of the PolyhedronData computational data collection, came into the picture.
As many of you may know, Mathematica‘s logo is a three-dimensional polyhedron affectionately called “Spikey.” In its original (Version 1) form, Spikey consisted of the spiked solid obtained from an icosahedron (the regular 20-faced solid that is one of the five Platonic solids) with regular tetrahedra (triangular pyramids) affixed to its faces.
 1
1
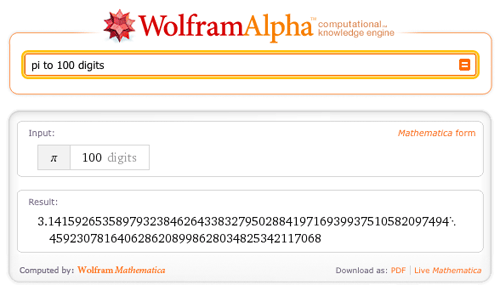
Some of you have asked whether you’ll be able to use Wolfram|Alpha for challenging math. Of course!
Remember your old friend pi?